The Analysis of the Real
Prof. Uri Hadar
Department of Psychology
Tel Aviv University
Ramat Aviv 69978
Israel
Short title: The Real
February 2009
Introduction
The order or the register of the Real is probably the most elusive of the Lacanian orders: On one hand, the term 'real' implies something concrete, perhaps even tangible. On the other hand, it is next to impossible to describe an actual experience that is Real or 'of the Real'. In the words of Lacan (1982) "One thing that is striking is that in analysis there is an entire element of the real of the subject that escapes us. . . There is something that brings the limits of analysis into play, and it involves the relation of the subject to the real". The Real order of experience is said to evade pinpointing by virtue of refusing symbolic representation. "What does not come to light in the symbolic appears in the real" (1966, p. 388). Since all experiential 'pinpointing' is necessarily symbolic- we need to represent experience in order to make it sufficiently compact to be 'pinpointable'- and since symbolic representation divorces an experience from its Real underpinning, one usually talks about the Real in terms of the lost object. The set of experiences that concern the sense that something has been there at some time in the past, or on some other level of the present, but can not be recovered.
There are a number of experiential phenomena that are cited as the marks of the Real or the points at which the subject makes contact with the Real. One such phenomenon is repetition compulsion, when the Real is said to be "that which always returns to the same place" (Lacan, 1978, p. 49). Another approach of the Real appears in a number of readings of Freud's case studies, when Lacan locates the source of terrifying hallucinations. Indeed, Lacan makes an explicit reference to the Real in the case of anxiety, of which he says that it has its object in the Real. Like with the other experiential phenomena, anxiety is not in and of itself Real, but it refers to the Real in the most direct manner afforded by articulable experience. Lacan's characterization of anxiety contrasts with popular formulations, which view anxiety as an affect that lacks an object- unlike fear, which is said to be directly associated with an object. Lacan ascribes an object to anxiety as well, but this 'object' is something that does not exist in any simple way: it may have existed- or may have been existing- but can not be circumscribed. Lacan formulates this in different ways, for example, “Anxiety sustains this relationship of not being without an object subject to the reservation that this is not to say nor to be able to say, as we could for something different, what object is involved” (Lacan, 2001; Chap X, p.1). The object of anxiety is neither Symbolic nor Imaginary, since a clear representational existence would have allowed us to specify it. One therefore says that the object of anxiety is Real: it is represented in both the Symbolic and the Imaginary as that which marks their lack, their residue, namely, the object petit ‘a’. “… this object ‘a’ is the one which is at stake everywhere Freud speaks about object when anxiety is involved” (Lacan, 2001; Chap. III, p. 10). Anxiety, then, is a major- if not the only- window to the Real. This is the revolutionary discovery of Seminar X (Lacan, 2001). In later formulations, Lacan extended the formulae of Seminar X to the notion of the symptom, construed as the limit of the symbolic in the Real. The symptom itself is, of course (like in all earlier formulations) Symbolic, but it marks a point of which there is no beyond. The place where the Symbolic links up or touches upon the Real (Lacan, 1974-1975).
The present article undertakes an analysis of the Real. One may feel that it is presumptuous to call 'analysis' the investigation of an order that clearly refuses constructive depiction. An order that, so far, has been discussed primarily in a manner that is circumscribed, perhaps even circumlocutionary. A manner that sets a context but steers clear of analytic definitions. Fortunately, we have Lacan's own depiction of 'analysis' to our rescue here: Lacan likes the use of the term in its entire psycho-mathematical range. His use refers, sometimes, both to psychoanalytic and to mathematical discourse, and suggests a link between them (Lacan, 1972). Such confluence of psycho-mathematical ideas offers me the blueprint with which to approach the Real in the present article, that is, to carry out a discussion that lays out the span of a psycho-mathematical analysis. I shall construe the Real by analogy to the mathematical analysis of real numbers, namely, by analogy to the manner in which mathematicians treat the formation of the continuum by numbers. My argument will be that the Real is analogous in status to irrational numbers, so one can approach the Real by analogy to the mathematical construction of irrational numbers. Specifically, I shall show that one way of characterizing irrational numbers is analogous to the Symbolic (de)construction of the Real, while another strategy of defining irrational numbers is analogous to Imaginary approximations (of the Real). Finally, I shall examine a few clinical and pseudo-clinical materials in the light of the present formulations. But first, let me discuss some pre-suppositions about my reading of Lacan that are necessary in order to justify the present approach (or at least in order to be clear about how justifying this approach can fail). It is my position that other readings of Lacan are possible and I have no claim for a true understanding of Lacan's intentions. In fact, all I wish to do in the present article is to offer a constructive account of the Real that is Lacanian in the minimal sense of giving a relatively consistent reading to some well-known aphorisms of Lacan (that are related to the order of the Real). It is not my ambition to offer a reading of everything Lacan has said about the Real, but only of a subset that my reading is capable of depicting consistently.
Pre-suppositions
The present reading of Lacan's Real takes a post Kantian, or post-post Kantian position in a sense that is pertinent to the specifics of this analysis and according to which there is no direct access to reality. All contact with the thing in itself is mediated by representational systems, be they Symbolic or Imaginary, which project their internal logic and structure upon the related concepts, precepts and inferences[1]. One implication of this position- and none of a big deal- is that the Real does not and can not relate to the thing itself (as opposed to the thing for a subject). Everything that may be represented or spoken is always already for a subject. The Real, in that sense, is a property of all representation that determines its nature as a representation. Thus, a representational system- and let me not dwell much on this term, 'system'- is representational inasmuch as it points to something that is outside of itself. This property is inherent in the concept of representation, which is not, again, a big deal. However, every representational system must encapsulate in its logic and structure this very property. A representational system constitutes itself as representational inasmuch as it announces its representationality. Its being a representation. And it does so by indicating that there is something in the world that is outside of the representational system and to which it refers, of which it is representational. Reality, in that sense, is (merely) a property of representational systems. In words that could, in my reading, be Lacan's, representational systems are Moebius strips which generate an externality without ever being able to posit such externality other than as their internal property. Now, in my reading, the Real is the externality of both the Symbolic and the Imaginary. It is as though the Symbolic and the Imaginary are linked in such a way that the 'things' of which they are representational are the same. Another way of saying this is that the Symbolic and the Imaginary point in the same direction when they implicate an externality, and this direction is called the Real. This reading of Lacan is best articulated in his latter texts as, for example, in the following: "The symbolically real isn't the really symbolic. The really symbolic is the symbolic included in the real, which very well has a name – it's called lying. The symbolically real, being that part of the real that's implied in the interior of the symbolic, is anxiety. The symptom is real. It's even the only truly real thing, that is, the only thing that holds onto a meaning in the real. That's why psychoanalysis can, if given the chance, intervene symbolically to dissolve it in the real." (Lacan 1974-1975).
One notes here something that should have been clear from reading Lacan, but somehow is never being said explicitly, namely, that the order of the Real is different from the orders of the Symbolic and the Imaginary. Xxx עד כאן The latter two may be construed as representational systems- a term Lacan would probably not have liked- while the Real can not. Instead, the Real is indicated in both systems by those properties that determine their nature as representations. This implies that we can understand or investigate the Real by examining the manner in which the Symbolic and the Imaginary create their externality (where this externality is construed as an internal property). I assume, then, that the Symbolic and the Imaginary act to posit their externality in relation to themselves and, moreover, that they posit it in the same 'place': the Real. I think that it is easy to infer from the above formulations that the Real, the Imaginary and the Symbolic are inextricably linked by a tie that could be called 'Boromean' in the sense of radically subverting the ability to always determine what is inside or outside each of these orders. The three orders do not occupy connected regions, but are rather inter-dispersed among each other. These formal or structural properties are the ones that inform the present analysis.
Mathemic analysis
The word 'analysis' in the idiom above- in a context that is mathematic in some non-trivial manner- may be understood in two ways. In mathematics proper, analysis- by contrast to algebra- refers to the investigation of functions that are continuous at least in parts. However, this is not the way that Lacan uses this idiom. For Lacan (1972; 1980), mathemic analysis refers to the manner in which recourse to mathematical concepts may promote psychoanalytic investigations. The term Matheme refers to this stitching together of mathematical-psychoanalytic formulations (Miller, 1978). Now, the point that I wish to make here- like before (Hadar, 2005)- is that, in my reading, Lacan's use of mathematics is not like in the sciences, where mathematics acts to render thematic concepts more accurate or more rigorous. Lacan's later writings repeatedly stressed that psychoanalysis- always already- comes as rigorous as it may ever become. The point of Mathemizing is to address certain end point of the Symbolic order, namely, those where formality is maximized. 'Formality', in that sense, is simply the lack of influence of form on content or, in the terms of Lacan (1966), the extent to which the signified is free of the penetration of the signifier. In that sense, the importance of Mathemes (and the related text) is in showing that the 'pure' symbolic entities of mathematics and logic are not above psychoanalysis: they lend themselves to psychoanalytic treatment, like every other product of the mind. Of course, one can say this in the language of psychoanalysis (rather than in the language of methodology): Mathematical formulations also originate in primary processes whose nature may be articulated by marking the many loci at which mathematic-like symbols cross psychoanalytic concepts.
Consider, for example, the basic exposition of the manner in which language (the signifier) splits the subject (Lacan, 1977a). Here, the exposition starts with the laying out of symbols in the manner in which the division of large numbers is taught in French primary schools: The symbol of the signifier is divided by that of the subject, yielding another signifier, together with a 'remainder', which marks the irreducibility of the subject to the signifier. The main gain from using this procedure is in creating the image of reducing a whole to some parts which make it up in a systematic way and whose logic is graspable, unlike that of the whole. The secondary gain concerns the essential in-divisibility of the whole, associated with the notion of remainder, but this indivisibility is precisely the one that emerges from the process of dividing. Put differently, the remainder shows that the Symbolic order does not exhaust all mental processes (even if it offers their best approximation). In the subjective division of signifiers, the latter reflexively divide the subject so that the subject itself becomes 'divided' or split. Now, the formalism of the process of division is used here as a poetic device, a Matheme. Despite of the use of the visual format of division, as well as the terms 'division' and 'remainder', the diagrammatic procedure that shows the symbolic division of the subject by the signifier has very little to do with the literality of mathematical division. Instead, it acts metaphorically (as much as mathematics is concerned) in order to formulate the basic influence of language and symbolization on the way we position ourselves in the world. Indeed, the diagrammatic exposition does this very effectively and, at the same time, offers two added values: One concerns the juxtaposition of a mathematical procedure and a psychoanalytic statement, showing possible implications of the fact that desire is channeled ('cathected', if one so wishes) in symbolic modes. The other added value concerns the aesthetics of Symbolic economy, of brevity (with its mnemonic profits). Of course, economy of articulation is poetic as much as mathematic. Mathematics becomes here psychological more than the reverse.
Principles of real analysis
In mathematics, the fundamental object of real analysis is the understanding of what continuity implies and how it forms. Its paradigmatic theme is the manner in which the numerical continuum- also referred to as the axis of real numbers- can be formalized. Analysis progresses here, crudely, in the following manner. Natural numbers (1,2,3…) are the mathematical objects that are most easily grasped by the intuitive mind. As a set, it is infinite, but its infinity feels like it is imaginable, not terribly mind boggling. The first extension of natural numbers towards real numbers involves adding negative numbers so as to create the set of integers. Both natural numbers and integers are, of course, infinite sets, but the magnitude of their infinity is the same in the sense that it is possible to match one set onto the other in a one-to-one manner. For example, we can match all positive numbers with odd numbers (Nn=2n-1; n>0) and all negative numbers with even numbers (Nn= -2n; n<0). We see here a property that distinguishes infinite sets from finite sets: A finite set can never be equivalent in number of members to a subset of it, while infinite sets can. Thus, a subset of integers such as natural numbers can have the ‘same number’ of objects as its super-ordinate set. Integers extend to rational numbers by adding all numbers that are quotients of integers (k/n where k and n are integers). Rational numbers have the property that between every two integers there is an infinite number of rational numbers. Moreover, between every two rational numbers there is an infinite number of rational numbers, so the set of rational numbers is very crowded indeed (infinitesimally so). Despite its density, the infinity of rational numbers is of the same order as that of natural numbers (constructing a one-to-one match is a bit elaborate here, so I shall skip it, but it may be found in many textbooks of set or number theory). Now, it is easy to show that rational numbers do not create the whole continuum, even only by showing that Pi ( π – the ratio between the circumference and the diameter of a circle) and Ln (the natural logarithm) are not rational. Since the sum of each of them with any rational number is also not rational, we have at least as many irrational numbers as rational numbers. Since this procedure of adding rational numbers to all sums of rational+irrational numbers can be repeated infinitely, the number of irrational numbers at least squares the number of rational numbers, but are these infinities of the same magnitude? And how do irrational numbers add up to create the continuum? Well, the continuum is simply defined as the set of all real numbers, which is the union of the sets of rational and irrational numbers. Since the properties of rationality and irrationality are logically complementary, one can say that every real number is either rational or irrational. Of course, irrational numbers are very many (in fact, of a different magnitude of infinity than rational numbers), but we know very little about them, except for a few of them that may be rationalized algebraically or geometrically (such as Ln and π).
Now, I suggest that, in the present context of the relationships between the Symbolic and the Real, rational numbers can be compared to the Symbolic order, and this for a number of reasons. Firstly, the property that defines them- divisibility- also defines the relationship between signifiers and the subject (see 'Mathemic Analysis' above) which, in turn, constitutes the Symbolic order. Indeed, the Symbolic order can be defined as the products of the division of signifiers by the subject (and, of course, as a consequence, the division of the subject by the signifiers). Secondly, and more crucially in the present context, the manner in which rational numbers approximate irrational numbers is suggestive of the manner in which the Symbolic positions the Real. This happens for the following reasons. There is a basic theorem of real analysis whereby every real number may be presented as the limit of an infinite series of rational numbers. Thus, an irrational number can never be represented by numerals (only rational numbers can), but it can be given a name (for example, Pi and Ln) and, more importantly, for every irrational number IR, one can always find a rational number Rn that is arbitrarily close to it or, to state this more clearly, every IR has a rational number Rn that is infinitesimally close to it (this is one of the implications of there being an infinite series whose limit is IR). We may summarize this aphoristically by saying that one can not know irrational numbers from within, but one can know them from without.
By analogy to the approximation of irrational numbers by (infinite) series of rational numbers, an element of the Real can be approximated by Symbolic means. Of course, like Pi and Ln, Real elements can be given names (for example, the term Real itself or the term 'objet petit a'). This is not very exciting and it conveys nothing in and of itself. But there are also various texts or bits of texts that approximate the Real (by Symbolic means). These are series of concepts that portray the Real not by naming, but by creating a certain conceptual dynamics that leads in a certain direction. One may extrapolate from these concepts to something that is of a different nature, which 'something' marks the Real. I illustrate such approximations in the next section.
The description of irrational numbers as limits of infinite series of rational numbers is but one strategy that describes them. Another strategy, called 'Dedekind cuts', describes irrational numbers in a very different manner. Instead of setting out from discrete elements (rational numbers) and defining the continuum as a set of infinite series (of rational numbers), the strategy of Dedekind cuts is to set out from the continuum and create a procedure that defines a particular element in this continuum. This procedure draws upon the analogy to the act of cutting, as its name indicates (and this would probably have been significant for Lacan if he ever discussed Dedekind). One may start with the intuition that when you cut something with an infinitely thin 'knife' you do not lose anything, but rather separate between two segments at a particular point: the point of the cut. Cutting, in that sense, separates a singular whole into two disjoint parts, where only one part can contain the point of the cut (this originates from the logic that every point, by being a point, appears only once). Every number n is defined here by the two sets that it serves to separate, N1 and N2, which are denoted by the couplet (N1,N2), where N1 contains all real numbers from — to n (not inclusive), and N2 contains all numbers from n (inclusive) to +. Now, since Dedekind's procedure sets out from the real continuum, it needs not show that every number- rational or irrational- is pointable by this procedure. Unlike with the rational approximation procedure, every number has been there to start with. Instead it is necessary to show that one can define the ordinary arithmetical operations on these sets (of separated sets), and that these definitions satisfy everything we know about the arithmetical operations on numbers. And indeed, Dedekind managed to show this in a full and comprehensive way.
Like in creating a number by cutting, an element of the Real can be positioned through an Imaginary cut. Here, just like in the Symbolic operation of division, the most basic consequence of cutting is that of separating in the Imaginary the domain of the subject from that of the other. Both the subject and the other lend themselves, within limits, to Imaginary and Symbolic representation, but the cut that separates them- in each concrete instance- is not. Instead, and by remarkable analogy to the Dedekind cut, the point of the Real that is asserted by the cut is marked as different: in the former case, it determines a specific number, while in the latter case it creates an object petit 'a'. The ‘a’, in that sense, represents for the subject the other as Real. This, in turn, may fall on either the side of the subject or the side of the other. If the object petit 'a' falls on the side of the other it generates desire, while if it falls on the side of the subject it generates anxiety. Many of the neurotic styles may be construed as cutting performances that aim to locate the 'a' on one side rather than the other. More often than not, these performances aspire to situate the 'a' in the domain of the other, thus constructing the subject as a desiring entity, rather than an anxious one. Some of these strategies may appear as mechanisms of defense. I can sum this up by saying that, in the Imaginary, the Real consists of the various cuts that separate subject from other. These cuts are marked by petit 'a's.
It is clear from the above discussion that approximation of the Real by the means of iterative divisions (that create infinite series of signifiers) is Symbolic, while marking the Real by the means of cutting through surfaces and bodies is Imaginary.[2] We have here, post-partum, a definition of the Symbolic and the Imaginary through the different ways in which they locate the Real. This definitional procedure is very different from the one that has commonly been used in explicating Lacan, where the Symbolic is said to be essentially linguistic, while the Imaginary is essentially visual. It is of much interest to show the ways in which these two kinds of definitional strategies act and inter-act, but such an exposition is beyond the scope the present paper.
Symbolic approximations of the Real
Lacan often described Symbolic approximations of the Real as asymptotic (e.g., Lacan, 2001). In an attempt to capture the Real- marked by the locus of the petit 'a'- the subject enters a dynamics of both repetition and gradual magnification. This dynamics tends to show the same pattern: In claiming the Real- that Real which the subject somehow conceives of as totally outside of the Symbolic- one enters upon a series of actions that have the common denominator of being directed at the petit 'a', but require increasing magnitudes in order to manage the eternal elusion of anything that is experientially of a different order (real). Indeed, the ultimate experiences in this vein- those of intense awe (trauma), mystic experiences or those of intense infatuation- get usually disconnected from any reality and remain wildly symbolic, engendering endless repetitions of the same sequences of images and emotions. I want to illustrate this dynamics with three kinds of phenomena, one relating to the paradigmatic case of repetition compulsion, as in compulsive disorders, one relating to the fear of a mystic failure to materialize (causing a repeated avoidance to take symbolic loans of the real) and one concerning Zizek's portrayal of the psychology of the consumer in post capitalism.
Consider the person who repeatedly checks- whatever- say, the closure of the main door in her home. What prevents this person from trusting her mnemonic evidence that the door is closed? It is not a lack of memory- this is present with crystal clarity- but the experience of this memory as not-real-enough. We all have the knowledge that our memory is entirely symbolic, but most of us are reasonably happy in our symbolic existence. It works fine for us. We still need to unlock the door on return. The compulsive person, however, needs, indeed, demands that closure be entirely real, entirely non-representational. The repeated frustration of this demand is what sends her to a frenzy of checking. By the nth time, she already knows that the kind of certainty she demands is inherently un-attainable and probably paradoxical, but she can not stand it. It is the Real that plays hide and seek with her.
I have recently heard of a story that I particularly like as an illustration of compulsiveness in an inversed and delimited form. A man had been organizing a workshop in which he was due to act as director and for which a brochure was being produced. He had been writing a doctoral dissertation for a few years and, on the day the brochure was due out, belatedly, he was informed by phone that his dissertation had been approved by the supreme committee of the university. He phoned the secretary responsible for the brochure and told her of the good news and added that he may be able to appear in the brochure with his new title. He asked the secretary whether she could hold the production of the brochure for two more days until he would get a written confirmation of his doctoral degree. His secretary said the brochure had to, really, be produced on that day- they were already well behind schedule with the distribution of leaflets. The man phoned the doctoral office back and asked whether he was already entitled to use his new title. The answer was clear and positive, so the man phoned the secretary to tell her to insert his new title in the brochure. She asked the man whether he was quite certain- they had to avoid an embarrassment- and in return he asked her to hold on for just a couple of minutes more, while he ensured that the positive confirmation was indeed positive, one hundred percent. He phoned the doctoral office again and asked them whether they could fax him an interim letter confirming his eligibility for use of the title. The doctoral secretary said she will see what she could do. The story need not be pursued any further here: The kind of certainty that the man was determined to obtain- in his excitement- was simply not of the order in which the Symbolic acts. The Real again played cruel games.
Finally, consider Zizek's portrayal of consumerism in post-capitalist society. There are a number of illustrations that were given by Zizek in different lectures, but the best developed one is probably that of Coca Cola (for example, Zizek, 1999). Wherein originates the endless attraction of Coke? Zizek (ibid) ascribes it to the way in which “Coke functions as the direct embodiment of "IT", the pure surplus of enjoyment over standard satisfactions” (P. 1). This surplus does not originate in any possible use value: Coke does not quench thirst any better than other drinks- in fact, much worse, judged by the fact that its fans always find themselves wanting more of it, still thirsty, as it were; Coke is not tastier, its taste is always strangely unpleasant in the beginning (but marks it out as different from drinks that are immediately more pleasant). In fact, says Zizek, its attraction links up precisely with its lack of use value- in its having only a surplus value. “It is rather this very superfluous character that makes our thirst for Coke all the more insatiable”. Yet, this surplus enjoyment, this “IT”, is not something that Coke affords its drinker, for if it did, (s)he would not need to continue to consume. It is the promise of an “IT” that Coke manages to engender in its fan, not its fulfillment. The pure notion of it as obtainable. In the words of Zizek (ibid) “it’s ‘it’ precisely insofar as it’s never IT”. As a result, “every consumption [of Coke] opens up the desire for more”. Drinking, as it were, brings the drinker to the verge of the promised ‘it’ but leaves a gap, a lack, that is never satisfied and which is, indeed, insatiable. The result is a need-free rate of consumption.
The cental mechanism that supports the above dynamics is ascribed by Zizek (ibid) to a particular kind of displacement, from the Freudian ‘Id’ to the superego. Whilst the classical (or modern) ‘it’ is tied up- indeed, is synonymous- with the Id, where needs have their obvious biological reality, when displaced to the superego the need is transformed into an injunction. From the experience of thirst, the drive to drink is transformed into the imperative form of ‘drink X!’, ‘you must drink X’, or ‘only if you drink X you will fulfill your duty to enjoy’. This detaches drinking from its real motive and ties it up, instead, with a Real motive. The promised land of the pure, ultimate real. In terms of the present formulations, this form of promised satisfaction is Symbolic and the erroneous apprehension of it as leading to ‘something else’ generates consumerism in its insatiable form. The more determined one is to get hold of the thing itself, to have the experience of ‘that’s it’, the more one falls into the grip of the virtual, of pure exchange value, of absolute Symbolism. In these cases, the Real plays expensive games.
Imaginary approximations of the Real
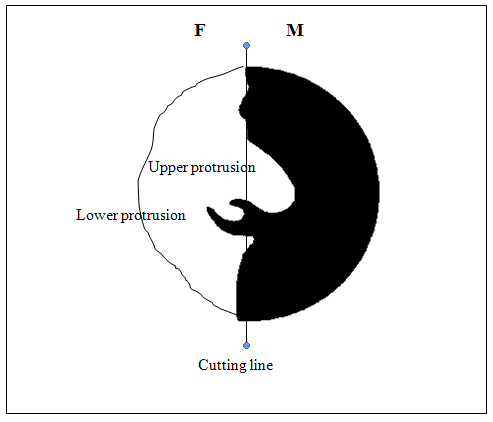
As said earlier, the Imaginary act that marks the Real is that of the cut. The cut inserts the petit 'a' on one or the other of its sides- that of the subject or that of the other. If 'a' appears in the field of the other, it engenders or ’causes' (Lacan (1999) insists on a causal relation here, Chapter VIII, p 3) desire, while if it appears on the side of the subject it causes anxiety. Inasmuch as Lacan considers anxiety to be prior to desire- here again by force of a ‘logical’ necessity, as he insists (Lacan, 1999; Chapter XIII, p-5)- the cut (and with it, the objet ‘a’) tends to fall in the domain of the subject. Mental boundaries, in that sense, tend to define the subject rather than delineate the Other. According to this logic, the dynamics that defines the subject as the one with the boundary also defines her as the one who is anxious. Fortunately, the imaginary plurality of crevices and protrusions (see below and Figure 1) ensures that the other also has the local properties of subjecthood, in the form of something which, the subject imagines, belongs now with the Other, but has originally been hers. For precisely the same reason- the plurality of protrusions and crevices- the subject has also got the properties of otherhood in the form of parts that, she imagines, have belonged with the Other but are now in possession of the subject. In that sense, the dynamics of subjecthood and otherness concerns the imaginary, asymmetric economy of objects ‘a’.
The above analysis construes such classical psychoanalytic processes as separations, individuations and the like- which distinguish subject from Other- as involving the performance of an imaginary cut. Those aspects of the relationship between the subject and the other which Lacan calls ‘specular’ are symmetric and form the basis for identifications. However, in every cut there are aspects that are non-specular and concern the economy of either-or. These build, topologically speaking, on crevices and protrusions in the domain of imaginary unity (see Figure 1). Lacan (2001; p. XIII- 10) expresses this powerfully: “on what side is this breast? On the side of the one who sucks or on the side of the one who is sucked? And after all, I am doing here nothing other than reminding you of something that effectively analytic theory was led to, namely to speak, I would not say indifferently, but with ambiguity in certain sentences, of the breast or of the mother, underlining of course that it is not the same thing. But has everything been said when the breast is qualified as a partial object? When I say amboceptor, I am underlinig that it is necessary to articulate the relationship of the maternal subject to the breast as that of suckling to the breast. The cut does not happen in the same place for the two; there are two cuts so distant that they even leave two residues for the two. Because the cutting of the cord for the child leaves separated from him droppings which are called the envelopes. This is homogeneous with himself and in continuity with his ectoderm and his endoderm.” In these non-specular regions, the cut does not separate neatly between subject and other, but it is exactly here that the Other is formed. In the Imaginary, then, the Other is formed of mental states that mark the asymmetry between subject and specular other.
Gender differences, or something like them, may be derived from the cut as some specific attributes of asymmetry or non-specularity that are determined by the pattern of crevices and protrusions through which the cut crosses (Figure 1). In the illustrated cut, F gains a lower protrusion and loses an upper one, while the reverse holds for M. This creates a complementary asymmetry of anxiety: gaining the lower protrusion ’causes' castration anxiety, while gaining the upper protrusion ’causes' separation anxiety. This asymmetry between castration and separation anxiety may act to define gender differences, but note that we have here an inversion of the classical notions that connect gender differences with anxieties: masculinity is associated with separation anxiety, while femininity engenders castration anxiety. In each case, anxiety is tied up not with the threat of losing a part of one’s body, but with owning a part that, in the Imaginary, is not one’s own. This is why circumcision, a metaphor of castration that is both Symbolic and Imaginary, constitutes men as subjects and generates their phallic desire. It’s not that the imagery related to circumcision is totally dependent upon its symbolic nature, but rather conversely, the Symbolic act of circumcision enacts the Imaginary constitution of the subject as Real. In the words of Lacan, “the phallus is more significant in human experience by its collapse, by its possibility of being a fallen object than by its presence: this is what designates the place of castration in the history of desire” (Lacan, 2001; Chapter XIII, P. 12). Of course, the above analysis holds only for separating Ms from Fs by the means of an idealized cut (represented in Figure 1 as the positioning and the straightness of the cut). The separation of M from M or F from F forms combinations of ‘a’ that are not well defined for gender at all. In that sense, the imaginary constitution of gender is only a local, idealized consequence of subject formation, and not a general property of separating subject from other.
Unlike in mathematical analysis, where the locus of the cut is arbitrarily glued to one of the two sets that it creates, in mental life the containment of the cut- of the ‘a’- is crucial. However, there is no prima facie preference as to where the ‘a’ will go. As a result, there is a continuous and a fairly irresolvable struggle to annex or ‘ennex’ the ‘a’ (if I am allowed a neologism). This is particularly vicious where the imaginary reigns with no symbolic mitigation, namely, where specularizations and identifications dominate. By illustration, I wish to delineate the manner in which these dynamisms act to shape psychoanalytic psychotherapy.
Lacan views the analyst’s construction of the psychoanalytic setting as based on the readiness of the analyst to own up to the position of 'a'. In Lacan’s definition of the four discourses, this marks the psychoanalytic discourse (Lacan, 2006) which, in that sense, reverses the hysterical discourse precisely by transcending the need to ennex ‘a’, to convert anxiety into desire. The analyst’s desire – by virtue of its special social positioning and careful staging- concerns allowing the anlysand to position ‘a’ with the analyst, even and especially when ‘a’ is equated with knowledge. This readiness to take the position of ‘a’ is said to be the desire of the analyst which, in turn, constitutes the transference (Lacan, 2002). This structural reversal of specularization, in and of itself, puts into action the therapeutic machinery of psychoanalysis. Firstly, it mitigates the destructive part of relating to the other. Secondly, it reduces anxiety through the mechanisms of containment which, in the present context, revolves around the containment of ‘a’. Thirdly, it generates the patient’s desire, initially in the form of transference desire (converted anxiety). Fourthly, it transforms the passage l’act, the self-destructive attempt to ominpotently determine the position of ‘a’, into an enactment, which always turns to the analyst, seeks her recognition. Eventually, all of the above allow the patient to situate the Real in those locations where it can be subjectified, namely, in the shortcomings of the Imaginary and the Symbolic.
Conclusion
My illustrations of the positioning of the Real in imaginary and symbolic modes concern cases that, in my judgment, locate the Real in a relatively clear fashion as that which mobilizes the subject in a forceful but non-declarative fashion. The Real never tells you where it is, but always, in any experience, somehow marks itself as that which is ‘it’. That which is full unto itself, the ultimate being. Real ghosts are everywhere, they saturate experience. We have here another property of the Real that is nicely illustrated in the analogy with mathematical analysis: irrational numbers, despite always escaping our intuition of what a number is, nevertheless are plentiful and everywhere. They fill in the gaps in our representational systems even, perhaps especially, when it is these systems themselves that create them (in way of gaps).
Both Symbolic and Imaginary submissions to the temptations and the terrors of the Real lead to untenable mental conditions: In the former case, this involves such ill routines as compulsion, frustration and addiction. In the latter case, this involves aggression, violence, fear and the like. The mission and the working of culture, it seems to me, is to enable the subject not to give in to these temptations and terrors. Culture acts to allow the subject to navigate the symbolic and the imaginary while withstanding the temptation to experience the ultimate, be it the ultimate knowledge (as in the case of mysticism), the ultimate being (as, for example, in the case of Kurz in "The heart of darkness"), or the ultimate pleasure (as, for example, in the case of Odysseus and the sirens). The attempt to reach beyond the representational is bound to lead to the collapse of higher mental functioning, as happened to Kurz, and had been avoided by Odysseus. And mysticisms, one need not be a scholar of culture to recall numerous exemplars here, tend to end up in inventing imaginary enemies who must be destroyed.
Of course, in this day and age, there is also the threat of virtuality, of attempting to radically smooth up the Real through virtual living, of a systematic avoidance of representational collapses, of a massive ignorance of existential gaps. The computerized home systems have tremendous representational capacities that may create the sense that all representation is up one's screen. The excessive time spent in front of the monitor by growing numbers of people often causes a marked reduction in the range of textures that traditionally extend the Imaginary and the Symbolic fields. This results in the narrowing of experience and, by consequence, the reduction of the sensual aspects of representational activity. Eventually, this is bound to engender various forms of dissociation from the body, from the senses, from sense. Suffering is clearly locally reduced in this manner, smoothed up, but probably only in order to resurface in more threatening and unmitigated forms. My rhetoric here should not be read as anti-technological: Inasmuch as spending time on the web accentuates the representationality of experience, inasmuch as people spend more time writing letters, messages, chatting; listening to music and looking at images and artifacts- computerized technology acts as a civilizing agent. However, as much as it may not be of good mind to presume that the Real offers itself for possession, pretending that it can be totally disposed of has its own pitfalls. While renders virtuality an object for clinical investigations, it seems to me that, on the whole, we are much more aware of its dangers than we are of the dangers of being, knowing or seizing the real.
References
Hadar, U. (2005) Algebraic perspectives on the symbolic constitution of gender in Lacan. Journal for Lacanian Studies, 3, 48-66.
Julien, P. (1994). Jacques Lacan's Return to Freud. New York: NY University Press.
Lacan, J. (1966). Écrits. Paris: Seuil.
Lacan, J. (1972). Seminar on 'The Purloined Letter'. Yale French Studies, 48, 39-72.
Lacan, J. (1974-1975). Le séminaire. Book 22: R.S.I. Ornicar?, 2-5.
Lacan, J. (1978). The four fundamental concepts of psycho-analysis (Alan Sheridan, Trans.). New York: W. W. Norton.
Lacan, J. (1982). Le symbolique, l'imaginaire et le réel. Bulletin de l'Association Freudienne, 1, 4-13.
Lacan, J. (2001) Seminar of Jacques Lacan, Book X: Anxiety (1962-1963, Translated by Ghallager, C). Eastbourne: Antony Rowe.
Lacan, J. (2002) Seminar of Jacques Lacan, Book VIII: Transference (1960-1961, Translated by Ghallager, C). Eastbourne: Antony Rowe.
Lacan, J. (2006) Seminar of Jacques Lacan, Book XVII: The Other Side of Psychoanalysis (1969-1970, translated by Grigg, R.). NY: WW Norton.
Miller, J-A. (1978). Suture (elements of the logic of the signifier). Screen, 4, 24-34.
Ronen, R. (2002) Representing the Real. Amsterdam: Rodopi.
Zizek, S. (1999) The superego and its acts. Website of the European Graduate School. http://www.egs.edu/faculty/zizek/zizek-superego-and-the-act-1999.html
Quotes (from seminar X)
…Many things can appear which are anomalous- this is not what makes us anxious. But if all of a sudden all norms are lacking, namely, what constitutes the lack- because the norm is correlative to the idea of lack- if all of a sudden it is not lacking… it is at that moment that anxiety begins. (P 12 of chapter III).
My reading of the bottom of page 6 of chapter 4 [‘petit a’ being the product of the subject looking at herself through the Other] is that ’through the mirror of the Other, the subject perceives herself as split, while the petit a’ appears in the domain of the other. This constitutes desire. If petit a' appears in the domain of the subject, this constitutes anxiety.
In explaining that anxiety in animals always involves the presence of the Other, even if this other is the experimenter, devising her tricks: (chapter V, p 5)- “For the dimension of the subject supposedly transparent in his own act of knowing, only begins with the coming into play of a specified object, which is the one that I try to circumscribe in the mirror stage, namely, in the image of one’s own body in so far as the subject in a jubilatory fashion has in effect the feeling of being before an object which makes him, the subject, transparent to himself”.
About the goal of psychotherapy (and effectively a definition of what it means to take a subject position (chapter IV, p 3)): “What the neurotic retreats from is not castration; it is making his own castration what is lacking to the Other, O, it is from making of his castration something positive which is the guarantee of this function of the Other… (P. 4) Dedicating his castration to this guarantee of the other is what the neurotic comes to a halt before…” In my reading this means that, at the crucial point of asserting herself in relation to the other, the subject has only her own castration with which to position herself. She has to give up the expectation of finding her true self in favor of asserting herself arbitrarily – making a choice- in relation to the Other.
VII-12: "That is how 'a' is made. [by a cut that subverts inside and outside- UH] It is made like that when any cut whatsoever is made, whether it is that of the cord, that of circumcision and some others still… There remains after this cut something comparable to the Moebius strip, something that does not have a specular image".
P.VII-13: "This is what is involved in the entry of 'a' into the world of the real, which it is only returning to".
VIII- 4: "… there where there is something which in discourse you articulate as being you, there where you say 'I', it is there, properly speaking, that at the level of the unconscious is situated 'a'".
VIII- 5: "… the anxiety of the other, his essential existence as subject with respect to this anxiety- this is what sadistic desire wants to make vibrate". [This involves locating 'a' in the domain of the other. It creates the desire of the (sadistic) subject at the same time as it creates the anxiety of the other].
IX-1: the diagrammatic juxtaposition of imaginary and symbolic approximations of the Real
X- 3: “Once it is known, once something of the Real comes to be known, there is something lost; and the surest way to approach this something lost is to conceive of it as a fragment of the body”.
X- Transference is construed as the conversion of anxiety into desire. This is achieved by securing the location of object ‘a’ with the Other (the therapist). In transference, the analyst is identified with object ‘a’. Therefore, in addition to converting anxiety into desire, the analyst also marks the place of (the lost) Real.
XII-4: “If this question [of counter-transference] is not simply not resolved, but finally has not even begun to be resolved, it is simply because there is not in analytic theory up to the present, I mean, up to this seminar precisely, any exact positioning of what desire is. … [the first step has been that] of teaching you to situate desire as distinct compared to demand… [I then specifically suggested to you] the identity, as I put it, of desire and the law. [in analytic doctrine] it is clear that what constitutes the substance of the law is the desire for the mother; inversely, what normatives desire itself, what situates it as desire, is what is called the law of the prohibition of incest.”
XII-6: “[the difference between the signifier and the trace] is what is demolished with the intervention of the real. The real, [by] referring the subject back to the trace, abolishes the subject also at the same time, for there is no subject except through the signifier, except through this passage to the signifier.”
XIII-5: “ ‘a’ is what remains irreducible in this total operation of the advent of the subject to the locus of the Other, and it is from this that it is going to take on its function” [ the ‘a’, that is].
…. “This remainder, therefore, in so far as it is the end, as one might say, of the subjective operation, this remainder, we recognize in it here structurally, in an analogy from calculation, the lost object; this is what we have to deal with, on the one hand in desire, on the other hand in anxiety. We have to deal with it in anxiety, logically, as one might say, before the moment that we deal with it in desire” [in my terms, we consider ‘a’ first from the perspective of the set to which the cut belongs and which marks the locus of the subject].
XIII- 10: “on what side is this breast? On the side of the one who sucks or on the side of the one who is sucked? And after all, I am doing here nothing other than reminding you of something that effectively analytic theory was led to, namely to speak, I would not say indifferently, but with ambiguity in certain sentences, of the breast or of the mother, underlining of course that it is not the same thing. But has everything been said when the breast is qualified as a partial object?
When I say amboceptor, I am underlinig that it is necessary to articulate the relationship of the maternal subject to the breast as that of suckling to the breast. The cut does not happen in the same place for the two; there are two cuts so distant that they even leave two residues for the two. Because the cutting of the cord for the child leaves separated from him droppings which are called the envelopes. This is homogeneous with himself and in continuity with his ectoderm and his endoderm.”
[1] Ronen (2002) presents a construction of the Real that is rather similar to the present one, albeit, with very different methods. However, in her reading, this construal runs contrary to the basic Kantian position. By consequence, Ronen is ready to assume a Real that is more independent of Symbolic and Imaginary constructs than the Real of the present article. Both Ronen's and the present approach are distinct and different from the Cartesian reading offered by Julien (1994).
[2] Chapter 9 of seminar X explicitly juxtaposes these two modes.